Example 1.1
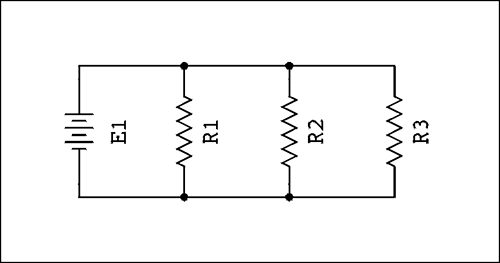
In Figure 1 E1 = 15 volts, E2 = 10 volts, V1 = 1.0 volt, V2 = 1.5 volts, what is V3 the voltage across R3?
Solution
E1 - V1 - V2 - V3 - E2 = 0 Solving for V3 gives
V3 = E1 - V1 - V2 - E2
V3 = 15 - 1.0 - 1.5 - 10 = 2.5 volts
You stand a better chance of getting the right answer if you don't take shortcuts but write it out this way.
Example 1.2
In Figure 1 if the current in R2 is 1.62 amps what is the current in; (a) the battery at E1, (b) R1, (c) R3, and (d) the battery at E2?Solution
The current is the same in all elements. All four answers are 1.62 amps.
Example 1.3
In the circuit of Figure 2 the battery current I is 127 mA, I2 is 33 mA, and I3 is 45 mA. What is I1?
Solution
I = I1 + I2 + I3 BACKSolving for I1 gives
I1 = I - I2 - I3
I1 = 127 - 33 - 45 = 49 mA.
Example 1.4
In the circuit of Figure 2 the battery voltage is 22.5 volts. What is the voltage across; (a) R1, (b) R2, and (c) R3?Solution
In a simple parallel circuit all voltages are the same. All three answers are 22.5 volts.
Example 1.5
A measurement on a 2 cell flashlight shows that the bulb current is 350 milliamps. What is the hot resistance of the bulb's filament? One cell produces 1.5 volts.Solution
We use the form of Ohm's law that will give us resistance.R = V / I If you used a calculator you likely got 8.571429 and maybe even more digits. These extra digits are not significant because the data we started with doesn't have that many digits. In this series of articles we will round off all answers to 3 digits. Leading zeros such as in the number 0.000359 are not significant. That number has 3 significant digits not 6.V = 2 x 1.5 volts = 3.0 volts
I = 0.350 amps (given)
R = 3.0 / 0.350 = 8.57 ohms
Example 1.6
You need a resistor that will drop 125 volts when the current is 26.6 milliamps. What is the value of this resistor in (a) ohms, and (b) kilohms?
Solution
You must work ohm's law in volts, ohms, and amps. But there is a shortcut. You can work it in volts, k ohms, and milliamps or even volts, Meg ohms and microamps. So let's work in k ohms and milliamps.R = V / I Round it off to 4.70 k ohms. The number of ohms will be a larger number than the number of k ohms. To convert to ohms multiply k ohms by 1000.R in k ohms = V in volts / I in milliamps
R = 125 V / 26.6 mA = 4.699248 k ohms.
R in ohms = R in k ohms x 1000 BACKR in ohms = 4.70 x 1000 = 4700 ohms.
Example 1.7
If you connect a 25 k ohm (same as kilohm) resistor across an 850 volt power supply, how much current will flow? Give your answer in m A (same as milliamps).Solution
So let's work in k ohms and mA.I in mA = V in volts / R in k ohms BACKI = 850 V / 25 k ohms = 34.0 mA
Example 1.8
Four resistors are connected in series. They are; 2.2 k ohm, 3.3 k ohm, 2.7 k ohm, and 2.4 k ohm. What is the equivalent resistance?Solution
The one equivalent resistor that could take the place of all four is the sum of the individual resistors.Req = R1 + R2 + R3 + R4 Notice that the sum is larger than the largest resistor. This is a good logical check for when you are working problems that you don't know the answer to and the result of a wrong answer could be a butned out circuit instesd of a low grade.Req = 2.2 k + 3.3 k + 2.7 k + 2.4 k = 10.6 k ohms
Example 1.9
Four resistors are connected in parallel. They are; 2.2 k ohm, 3.3 k ohm, 2.7 k ohm, and 2.4 k ohm. What is the equivalent resistance?Solution
The one equivalent resistor that could take the place of all four is one over the sum of the one overs individual resistors.Req = 1 / (1/R1 + 1/R2 + 1/R3 + 1/R4) BACKReq = 1 /(1/2.2 k + 1/ 3.3 k + 1/2.7 k + 1/2.4 k)
Req = 1/(0.000454545 + 0.000303030 + 0.000370370 + 0.000416666)
Req = 1/0.00154461 = 0.6474 k ohms = 647 ohms.
Example 1.10
In Figure 1 if R1 = 22 ohms, R2 = 18 ohms, and R3 = 12 ohms, what is the current through R1?Solution
In a series circuit the current is the same everywhere so to ask for the current in R1 is to ask for the current in any part of the circuit.We don't need to be concerned with the voltage across individual resistors. We can find the total resistance and the total voltage. That's all we need to calculate the current.
We begin by writing the voltage sum equation.
E1 = V1 + V2 + V3 + E2 Solving this equation for V1 + V2 + V3 gives,V1 + V2 + V3 = E1 - E2 The voltage across all three resistors is,V1 + V2 + V3 = 15 - 10 = 5 volts The sum of all three resistors is,RT = R1 + R2 + R3 = 22 ohms + 18 ohms + 12 ohms = 52 ohms So the current is the total voltage divided by the total resistance,I = VT / RT = 5 volts / 52 ohms = 0.0962 A = 96.2 mA BACK
Example 1.11
In Figure 2 if E1 = 6 volts, R1 = 22 ohms, R2 = 18 ohms, and R3 = 12 ohms, what is the battery current?Solution
The battery current is equal to the battery voltage divided by the equivalent resistance connected across it.Req = 1/(1/R1 + 1/R2 + 1/R3) BACKReq = 1/(1/22 + 1/18 + 1/12)
Req = 1/(0.04545 + 0.05555 + 0.08333)
Req = 1/0.18434
Req = 5.4246 ohms
I = V / R = 6 volts / 5.4246 ohms = 1.11 A
Example 1.12
In Figure 2 if the voltage across R1 is 77 volts, what is the voltage across R3?Solution
Figure 2 is a simple parallel circuit. The voltage across any element in a parallel circuit is the same as the voltage across any other element. Therefore the answer is 77 volts. Don't you wish they were all that easy.
Example 1.13
In Figure 1 if the current in R3 is 28 m A, what is the current in (a) R2, (b) R1?Solution
Figure 1 is a simple series circuit. In a series circuit the current through any element is the same as any other element. Therefore the answers are (a) 28 mA, and (b) 28 mA. Hmm, maybe they are all that easy.
Example 1-14
In Figure 1 the voltage across R1 is 1.9 volts, and the voltage across R2 is 0.8 volts. What is the voltage across R3?Solution
The voltage sums are written as follows,E1 = V1 + V2 + V3 + E2 Solving for V3 which is the voltage across R3 we have,V3 = E1 - E2 - V1 - V2 BACKV3 = 15 v - 10 v - 1.9 v - 0.8 v = 2.3 Volts.
Example 1-15
In Figure 2 The current in R1 is 1.3 Amps, the current in R2 is 2.5 Amps and the battery current is 10 Amps. What is the current in R3?Solution
Letting the current in R1 be I1 and so on we have,I = I1 + I2 + I3 Solving for I3 we have,I3 = I - I1 - I2 BACKI3 = 10 A - 1.3 A - 2.5 A = 6.2 A
Example 1-16
In Figure 1 the voltage drop across R1 is 1.25 volts, the drop across R2 is 1.50 volts, and the circuit current is 0.682 m A. What is the resistance of R3? Hint. This is what I call a two level problem. You have to make an initial calculation and then use the result of that calculation to calculate the final answer.Solution
To calculate the resistance of R3 we need to know the current through that resistor and the voltage across that particular resistor, not the voltage across some other resistor. Because this is a series circuit we are given the current as 0.682 mA. We need to calculate the voltage using voltage sums.E1 = V1 + V2 + V3 + E2 BACKV3 = E1 - E2 - V1 - V2
V3 = 15 v - 10 v - 1.25 v - 1.50 v = 2.25 Volts
R3 = V3 / I = 2.25 v / .682 mA = 3.30 k ohms.
Example 1.17
In the circuit of Figure 2 the battery current is 0.675 amps, the current in R2 is 0.15 amps, and the current in R3 is 0.275 amps. The resistance of R3 is 200 ohms. What is the resistance of R1? This is a three level problem.Solution
To calculate the resistance of R1 we need to know the current through R1 and the voltage across it. We are not given either one directly. We are given the current in R3 and its resistance. From this we can calculate the voltage across R3.V3 = I3 x R3 = 0.275 A x 200 ohms = 55 Volts. Because this is a parallel circuit the voltage across R3 is the same as all the other voltages so the voltage across R1 is 55 volts. Now for the current in R1.I1 = I - I2 - I3 = 0.675 A - 0.15 A - 0.275 A = 0.250 A The resistance of R1 is,R1 = V1 / I1 = 55 v / 0.25 A = 220 ohms BACK
Example 1.18
You want to connect a resistor substitution box across the 120 volt AC power line. Don't ask why, you just want to. The box uses 1 watt resistors. The values you can select are as follows15 ohm, 22, 33, 47, 68, 100, 150, 220, 330, 470, 680, 1 k ohm, 1.5 k, 2.2 k, 3.3 k, 4.7 k, 6.8 k, 10 k, 15 k, 22 k, 33 k, 47 k, 68 k, 100 k, 150 k, 220 k, 330 k, 470 k, 680 k, 1 Meg ohm, 1.5 Meg, 2.2 Meg, 3.3 Meg, 4.7 Meg, 6.8 Meg, and 10 Meg ohm.
What is the lowest value you can set the box to without burning out a resistor (exceeding 1 watt)?
Solution
Let's take the V squared over R formula and solve it for R as follows.P = V squared / R Don't ask me what a square volt is.R = V squared / P = (120 x 120) square volts / 1 W = 14400 ohms = 14.4 k ohms.
The two closest settings are 10 k ohms and 15 k ohms. If we set the resistance below 14.4 k ohms the power will exceed 1 watt. The setting to use is 15 k ohms. And that's my final answer.
Example 1.19
Express the following numbers in scientific notation.(a) 5280
(b) 26.48
(c) 0.0000036
(d) 525.8
(e) 0.0162
(f) 74890000
(g) 0.000000125
(h) 152000
(i) 9730000000
(j) 0.0000000000022
Solution
(a) 5280 = 5.28 x 103 = 5.28e3
(b) 26.48 = 2.648 x 101 = 2.648e1
(c) 0.0000036 = 3.6 x 10-6 = 3.6e-6
(d) 525.8 = 5.258 x 102 = 5.258e2
(e) 0.0162 = 1.62 x 10-2 = 1.62e-2
(f) 74890000 = 7.489 x 107 = 7.489e7
(g) 0.000000125 1.25 x 10-7 = 1.25e-7
(h) 152000 = 1.52 x 105 = 1.52e5
(i) 9730000000 = 9.73 x 109 = 9.73e9
(j) 0.0000000000022 = 2.2 x 10-12 = 2.2e-12
Example 1.20
Fix each of these numbers to be correctly expressed in scientific notation.(a) 47.0 x 103
(b) 0.025e-6
(c) 28.7 x 106
(d) 365 x 10-12
(e) 455e3
Solution
(a)47.0 x 103 (b)
Smaller, Larger
4.70 x 104
0.025 x 10-6 (c)
Larger, Smaller
2.5 x 10-8
28.7 x 106 (d)
Smaller, Larger
2.87 x 107
365 x 10-12 (e)
Smaller, Larger
3.65 x 10-10
455 x 103 BACK
Smaller, Larger
4.55 x 105
Example 1.21
Perform the following calculations and express the results in correct form.(a) 1/4.7 x 10-6
In scientific notation 1 = 1 x 100 (b) 2.5 x 10-3/4.7 x 103Mantissa = 1/4.7 = 0.212766
Exponent = 0 -(-6) = 6
1/4.7 x 10-6 = 0.212766 x 106
0.212766 x 106
Larger, Smaller
2.12766 x 105
Rounding off
2.13 x 105
M = 2.5/4.7 = 0.5319149 (c) 1.5 x 106 x 2.2 x 10-6E = -3 - (3) = -6
2.5 x 10-3/4.7 x 103 = 0.5319149 x 10-6
0.5319149 x 10-6
Larger, Smaller
5.319149 x 10-7
Rounding off
5.32 x 10-7
M = 1.5 x 2.2 = 3.3 This result can be expressed without writing the power of ten.E = 6 + (-6) = 0
1.5 x 106 x 2.2 x 10-6 = 3.3 x 100
1.5 x 106 x 2.2 x 10-6 = 3.3 (d) 1.2 x 103 + 8.2 x 102First we must make the powers of ten match.
1.2 x 103 + 0.82 x 103 Putting it back with its power of ten,1.2 + 0.82 = 2.02
2.02 x 103 BACK