For a verbal description click here.
Figure 1 Illustration of the Miller Effect.
Electrical Fundamentals.
Multi Grid Tubes.Odes to Tetra, Penta, and Hepta.
The triode was, and is, a pretty good tube but it does have a few problems. These problems were curable by inserting more grids into the tube. This gave rise to the Tetrode, 4 element tube, and the Pentode, 5 element tube. A special need in radio receiver design gave rise to the heptode, 7 element tube.Problems with the Triode.
The misbehavior of the Triode was attributed to the effect of plate to grid capacitance. There were two basic problems. These were 1) the miller effect and 2) oscillation.The Miller Effect.
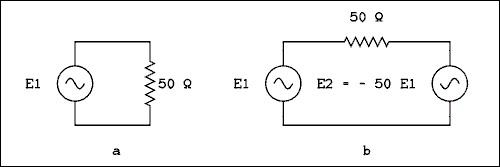
Because of the way a tube is constructed there is capacitance between the plate and grid. Because the tube inverts the signal the capacitance appears to be much bigger than it actually is. Lets explain it with resistors first. In Figure 1a an AC generator is driving a single resistor. In Figure 1b There is another generator in the circuit that always does the opposite of the one on the left but 50 times greater.

For a verbal description click here.
Figure 1 Illustration of the Miller Effect.
In Figure a suppose the generator is delivering 1 volt. The current is 1v / 50 ohms = 0.02 amps. In Figure b the voltage across the resistor may be found by summing the voltages around the loop. To do that we bring time to a stop so the AC becomes DC. The equation is,E1 - Vr + E2 = 0 The current through the resistor is 51 V / 50 ohms = 1.02 amps. The 1 volt generator doesn't know about the 50 volt generator on the other end of the 50 ohm resistor. It doesn't know anything, it's just a stupid generator. All it knows is that when it puts out 1 volt it has to deliver 1.02 amps so it thinks it's driving a 0.98 ohm resistor. The second generator has made the resistor look 51 times smaller than it actually is. If a capacitor were to be substituted for the resistor its reactance would look 51 times smaller and since capacitance is inversely proportional to reactance the capacitor will look 51 times bigger.1 - Vr + 50 = 0
Vr = 1 + 50 = 51 volts.
The effective input capacitance of a triode amplifier is given by,
Cinp = Cin + Cgp ( Av + 1 ) Where Cinp is the input capacitance of the amplifier, Cin is the tube manual value of input capacitance for the tube and Cgp is the given value of grid to plate capacitance.Example 5.1
In the previous chapter we calculated the gain of a 12AX7 amplifier to be 65.2. The tube manual gives the input capacitance of the 12AX7 to be 1.6 pf and grid to plate as 1.7 pf. What is the effective input capacitance of this amplifier.Solution
The Miller Effect is some what of a problem at audio frequencies. The reactance of 114 pf at 10 kc is 140 k ohms. The effective output resistance of the 12AX7 amplifier we have been working with is the parallel combination of the tubes plate resistance, the plate resistor, and the following grid resistor. That works out to 52 k ohms. The upper 3 dB frequency is where Xc = R. If this tube stage were followed by an identical one the cutoff frequency would be f = 1 / (2 pi R C ) = 1 / (2 pi 52 k 114 pf ) = 26.8 kc.
Oscillation.
When triodes are used as radio frequency RF amplifiers all Hell breaks loose. The phase shifts that result from the use of tuned circuits in the grid and plate cause the signal fed back through the plate to grid capacitance to be positive feedback. That spells oscillator. If that's what you wanted then your delighted. But if you wanted to amplify small signals to make them bigger you would not be a happy camper.There is such a thing as neutralization where in the circuit is arranged to feed signal back to the grid which is always opposite in phase and equal in amplitude to that fed back through the plate to grid capacitance. Neutralization is hard to adjust and increases circuit complexity. If there was a way to reduce the plate to grid capacitance...
The Tetrode.
Someone thought to place another grid between the control grid and plate. This grid would serve as an electrostatic shield to effectively reduce the capacitance. Just one problem, well, maybe two. The second grid severely reduce the gain of the tube unless it was placed at a high potential, equal to or even higher than the plate. That did solve the problem but it created another one.The problem was called secondary emission. What happens is when electrons strike the plate they are carrying a lot of kinetic energy. They give up that energy, in the form of heat, to the plate. The plate gets hot enough to begin emitting electrons. The impact of the incoming electrons helps to liberate others from the plate material. Think of them as being knocked loose. In a triode these secondary electrons have no place to go except back to the plate. It is the most positive thing in sight. In a pentode the screen grid is sitting there at a positive potential and because the plate voltage is going up and down with signal the screen grid may be, at that instant, more positive than the plate. The secondary electrons take off for the screen grid and ignore the plate. This takes away from plate current and adds to screen current. Both things are bad. But even worse is the negative resistance effect. There is a point where an increase in plate voltage will give a decrease in plate current. If you try to work that one out with ohm's law you get a negative value for resistance. I have observed this phenomenon myself in a laboratory experiment. This effect can be, and has been, used to make an oscillator but in an amplifier it leads to massive distortion. That's why there aren't any true tetrodes in your tube manual.
The Pentode.
So now there are three grids in our tube. You may think it's getting a little crowded in there but just wait until we get to the heptode. The third grid is called the suppressor grid or usually just the suppressor. It is usually placed at the same potential as the cathode. In some tubes it is internally connected to the cathode. The wires of the suppressor are widely spaced as compared to the control and screen grids so there is little effect on the high energy electrons coming from the cathode on their way to the plate. The secondary electrons are much lower energy and the suppressor is just enough to discourage them from going anywhere but back to the plate.So there we have the modern pentode tube. High gain, small Miller Effect, and low likelihood of oscillating.
Practical Circuit.
Like the triode the pentode can be connected as a resistance coupled amplifier. The tube manual circuit is shown below.

For a verbal description click here.
Figure 2 A pentode resistance coupled amplifier stage.
Once again the heater connections are assumed. The notation used here is consistent with the resistance coupled amplifier charts found in all tube manuals. You can design a pentode amplifier based on these charts and they will give you the gain but they don't say anything about Miller Effect.Small Signal Calculations.
The equivalent circuit and calculations are quite different from the triode amplifier. The circuit is shown in Figure 3 below.
For a verbal description click here.
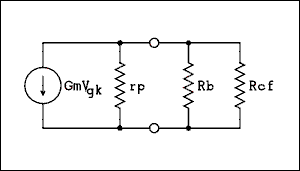
Figure 3 the small signal circuit of a pentode.
When you look up a pentode in a tube manual you will find the transconductance rather than the amplification factor. If you are curious you can calculate it very simply. The amplification factor of a pentode may be found by,mu = Gm rp Where mu is the amplification factor, Gm is the transconductance in mhos and rp is the plate resistance in ohms. The gain of a pentode amplifier may be calculated by,Av = Gm rp Rbc / ( rp + Rbc ) Where Rbc is the parallel combination of Rb and Rcf as follows.Rbc = Rb Rcf / (Rb + Rcf) If the cathode bypass capacitor is left off the gain equation becomes.Av = Gm rp Rbc / [ rp + Rbc + Rk ( Gm rp + 1 )] Where Gm is the transconductance of the tube, rp is the plate resistance Rk is the cathode resistor and Rbc is the parallel combination of Rb and Rcf as defined above.One note of caution. The transconductance of a pentode is very sensitive to screen grid voltage. For example, for the 6AU6 the lowest value for transconductance is given for a screen voltage of 100 volts. Yet the resistance coupled amplifier charts give values of Eg2 of around 40 volts. Obviously the value of Gm needs to be scaled down accordingly. The tube manual gives a graph of transconductance versus grid number 1 voltage for several values of grid number 2 voltage.
Example 5.2
A 6AU6 has a stated transconductance of 3900 micro mhos and a plate resistance of 0.5 meg ohms. These values are given for a g2 voltage of 100 volts. Now we look at the transfer function graphs and find the g1 voltage that gives 3900 micro mhos on the g2 = 100 volts curve. Now if we look at the same g1 voltage the point on the g2 = 50 volts curve gives a transconductance of 2200 micro mhos. What will be the gain of a resistance coupled amplifier using a 220 k ohm plate resistor and the following stage grid resistor of 470 k ohms. The cathode resistor is 1000 ohms. What is the gain of this amplifier (a) with a cathode bypass capacitor and (b) without a cathode bypass capacitor?Solution
Gain for an Unbypassed Cathode.
Resistance coupled amplifier charts don't give gain values for an amplifier with no cathode bypass capacitor. The approximation RL / Rk is so poor for tubes that it isn't worth fooling with. If you want to design a low distortion pentode amplifier and need to know the gain for an unbypassed cathode here is the procedure.1. Design a pentode amplifier from the resistance coupled amplifier charts in a tube manual.
2. Use the stated value of low distortion gain in the equation below to find the operating value of Gm.
Gm = Av ( rp + Rbc ) / ( rp Rbc ) 3. Use this value of Gm in the unbypassed equation to calculate the true gain.Example 5.3
The plate resistance of the pentode section of a 6U8, triode - pentode, is given as 0.4 meg ohms. The resistance coupled amplifier chart gives the following data.Ebb = 250 v
Rb = 270 k ohms
Rg2 = 820 k ohms
Rcf = 1 meg ohm
Rk = 1200 ohms
Ib = 0.72 mA
Ig2 = 0.26 mA
Ec1 = -1.0 v
Ec2 = 37 v
Eb = 55 v
Esig = 0.1 vAC
Eout = 22 vAC
Gain = 220
%Dist = 0.82 %
Esig(1) = 0.35 vAC
Eout = 63 vAC
Gain = 180
%Dist = 4.8%
(1) Taken at grid, g1, current of 1/8 microamp.
This is a lot more information than we need to work the problem but I thought I would give you the whole thing to give you an idea of what you can get from resistance coupled amplifier charts.
Calculate (a) the operating transconductance of the tube, (b) the gain without a cathode bypass capacitor.
Solution
The Miller Effect with Pentodes.
And you were thinking I would never get back to it. We have an example so lets work an example.Example 5.4
Using the full gain of the pentode section of a 6U8 above calculate the effective input capacitance. Capacitance values for a shielded tube are as follows. Cin = 5 pf. Cgp = 0.006 pf.Solution
From this calculation you can see that a pentode has at least two advantages over a triode. 1) it has more gain and 2) it has a much lower input capacitance. Pentodes do tend to produce more distortion than triodes which accounts for the popularity of the 12AX7. But in RF circuits you almost never see triodes.
The Heptode.
As the name implies the heptode has 7 elements, one cathode, 5 grids, and 1 plate. This tube is often called a pentagrid converter. Pentagrid as in 5 grids. It has one application, to serve as a mixer in radio receivers. (I have breadboarded and tested an audio voltage controlled amplifier using one of these tubes). For the gory details about these tubes I refer you to other pages on this site. Under the heading of "Radios" Simple Superhet, and under the heading "The All American 5" The Converter.
A foolish man dreams of wealth,
a rich man dreams of wisdom,
and a wise man dreams of tubes aglow all in a row.
This page last updated March 4, 2004.
Home