
undefined
undefined
HOME

Gothic Chess is a very fascinating Chess-variant that in my opinion,
along with Progressive Chess and Suicide Chess are the best Chess variants!
(And perhaps the best of these 3). It has been created by Ed Trice, who also has the patent for it.
If someone knows to play Chess it can learn to play Gothic Chess in 15 seconds
and if not, 2-3 minutes are enough to learn it, and have many hours of
enjoyment as it is a very fun game to play! It's "tempo" (the speed that the game
has) is higher than Chess and in fact the games in Gothic Chess are much shorter than
in Chess. In this page i will give the complete rules to play the game, some "statistics"-hints
for the values of the pieces and in the future i will write many commented games (by me and others), some amazing positions from games,
and some Mathematical facts for the game......
What follows are the complete rules for the game. As i've said even if you don't know Chess
you can learn to play Gothic Chess in 2-3 minutes. So don't be afraid by the
number of rules i have given! As i always try to be as accurate as possible,
i had tried to give all the rules that this game is apart from. But it's simplier than
it looks when reading my set of rules.
.......Starting board........
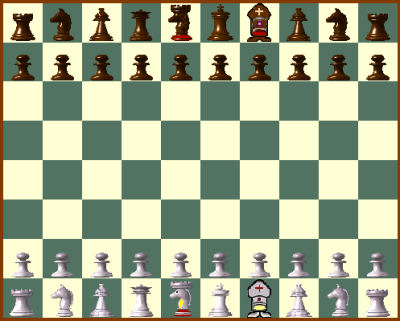
COMPLETE RULES for GOTHIC CHESS
- The board that G.C is played, is composed of an 10x8 grid of 80 equal squares alternately light (the 'white' squares) and dark (the 'black' squares).
The board is placed between the players in such a way that the near corner square to the right of both players is white.
- The board is consist of 10 files (vertical lines of squares) and 8 ranks (horizontal lines of squares).
The files are indicated by the letters (from left to right for White and from right to left for Black):
a,b,c,d,e,f,g,h,i,j. The ranks are indicated by the numbers (from bottom to top for White and from top to bottom for Black)
1,2,3,4,5,6,7,8. (So is concluded that every one of the 80 squares is called with a unique combination of a
latter and a number(for example: a6)).
- At the beginning of the game one player has 80 white coloured pieces
and the other has 80 black coloured pieces available for playing the game, altough most of them will never be used. White and black respectively have the following
pieces:
1 King, 11 Queens, 12 Rooks, 12 Knights, 12 Bishops, 11 Archbishops, 11 Chancellors, 10 Pawns.
These pieces have the following notation: K for the King, Q for the Queen,
C for the Chancellor, A for the Archbishop, K for the Knight, B for the Bishop,
R for the Rook and P for the Pawn.(The movement of each piece will follow later...)
!!!!!NOTE: To avoid possible misunderstandings it has to be clear that
the game of Gothic Chess it is played at the beginning with 20 pieces for each player.
10 Pawns and 10 other pieces that i specify at rule 4. And in the process of the game this number decreases
as captures are being involved. I refer that every player has 80 pieces AVAILABLE, in
order to cover all possible game continuations.
- 20 of these pieces for each player are placed on predefined positions in the
following way:
The white Pawns are placed to a2,b2,...,i2,j2 while the
black at a7,b7,...,i7,j7.

Two white Rooks at a1,j1 while two black at
a8,j8.

Two white Knights at b1,i1 while two black at b8,i8.

Two white Bishops at c1,h1 while two black at c8,h8.

A white Queen at d1 while a black at d8.

A white Chancellor at e1 while a black at e8.

A white Archbishop at g1 while a black at g8.

The white King at f1 while the black at f8.

- Every piece can occupy every square and in any square there can be
only one piece. Exception here is that Pawns can't move to the
a1,b1,...,i1,j1,a8,b8,...,i8,j8 and when a Pawn reaches any of these squares,
the player has the right to promote the Pawn to any other piece
he want, except King.The promotion is simple. When the Pawn reaches
the appropriate square it's being replaced by any other piece the player wants
as part of the same move and the effect of the promotion is
immediate.
- White player (that with the white pieces) playes first and then
the turn alternates from one player to the other. A move is mandatory
and if a player can't make a move the game is declared as a draw, if there is not any check by anyone.
- The game is ended either by:
a checkmate of any player, or
by a draw, or by resign of any player.
- The game is drawn when:
-
The player to move has no legal move and his king is not in check.
The game is said to end in "stalemate".
- The game is drawn when a position has arisen, in which neither
player can checkmate the opponent's king with any series of
legal moves.
- The game is drawn upon agreement between the two players
during the game.
- The game is drawn, upon a correct claim by the player having the move,
when the same position, for at least the third time (not necessarily
by sequential repetition of moves):
- is about to appear, if he first writes his move on his scoresheet
and declares to the arbiter his intention to make this move, or
- has just appeared, and the player claiming the draw has the move.
Positions as in (a) and (b) are considered the same, if the same player has the move, pieces of the same kind and colour occupy the same squares, and the possible moves of all the pieces of both players are the same.
Positions are not the same if a pawn that could have been captured en passant can no longer be captured or if the right to castle has been changed temporarily or permanently.
- The game is drawn, upon a correct claim by the player having the move, if:
- he writes on his scoresheet, and declares to the arbiter
his intention to make a move which shall result in the last
50 moves having been made by
each player without the movement of any pawn and without the capture of any piece, or
- the last 50 consecutive moves have been made by each
player without the movement of any pawn
and without the capture of any piece.
Here should be noted that perhaps there are special cases, where
for example we have KAKNN or KCKQ or KQKA or KQKNN or KNNKP or other...
and the one side has a forced mate but it is on more than 50 moves.
In the past there were special cases for Chess that allowed mate in more than 50 moves by each player, even if the above rules
were violated. But now there have been abandoned. I will ask
from game's inventor what is really exist for Gothic Chess and i will
report it here.
-
Movement of the pieces:
- Here a general rule exists: No piece can move in a way that it will
expose or leave its King in check!
- King
The King has 2 type of movements:
1.Moving to any adjoining square not attacked by one
or more of the opponent's pieces.
2.Castling (More on this later)
- Bishop
The Bishop may move to any square along a "free" diagonal on which stands, by not
making any leaps over other pieces. That's the meaning of free....
- Rook
The rook may move to any square along the "free" file or the "free" rank on which it stands.
Also it has the extra-move of castling (More on this later....)
- Knight
The knight may move to one of the squares nearest to that
on which it stands but not on the same rank, file or diagonal.
- Pawn
The pawn may move forward to the unoccupied square immediately
in front of it on the same file OR if it is on its starting move,
it may (alternatively) advance two squares along the same file
provided both squares are unoccupied OR the pawn may move to
a square occupied by an opponent's piece, which is
diagonally in front of it on an adjacent file, capturing that piece.
Also there is a special move called "en passant". More on this later...
- Queen
The queen combines the moves of the Bishop and the Rook and she can
use one per move.
- Chancellor
The Chancellor combines the moves of the Knight and the Rook and it can
use one per move.
- Archbishop
The Arschbishop combines the moves of the Knight and the Bishop and it can
use one per move.
-
In each of the above movements except Pawns,
that the matter has been specified,
when the move of the piece ends in a square occupied by an opponent's
piece, then the opponents piece is captured and removed from the game, while the moving
piece is placed on its place.
-
Castle.
- Castle is a special move of the King and a Rook. Is executed
as follows: The King is transferred from its original square
three squares towards the Rook, then that Rook is transferred
to the square, next to the King in the opposite side
in which that Rook was before, if King defines each side.
- Castling is illegal, for the rest of the game(for a specific side):
- if the king has already moved, or
- with a rook that has already moved.
- Castling is prevented temporarily:
- if the square on which the king stands, or the square which it must cross, or the square which it is to occupy, is attacked by one or more of the opponent's pieces.
- if there is any piece between the king and the rook with which castling is to be effected.
- A pawn attacking a square crossed by an opponent's pawn which has advanced two squares in one move from its original square may capture this opponent's pawn as though the latter had been moved only one square. This capture may only be made on the move following this advance and is called an "en passant" capture.
Actually these are not the complete rules for playing Gothic Chess,
but just the basic rules for having a correct play between 2 players.
For most technical rules like "The act of moving the pieces, touching of the pieces...", "The Gothic Chess clock procedures"
you should read FIDE laws of Chess that should be the same with these of this game.
PIECE VALUES
In order to play someone a good Gothic Chess game he needs to
get a feeling of how strong are these 2 new pieces(assuming he knows the other "Chess" pieces),
but also if the strength of the other "Chess normal" pieces remains the same in this game......
For doing this there are many possible ways. One is to play many games and get a feeling of
the strength of the pieces. Other way is to define some measuring procedures that would give us
an approximation of the pieces strength.
So lets define such a way:
DEFINITION-1: The strength of any piece(except Pawns and King)
has a linear relation with the probability of having a safe check to the King,
when the King and the piece are placed randomly on the 10x8 board alone(counting and the illegal positions). Safe check is a check that
the piece gives, without the King being able to capture it.
So now with the above definition lets calculate the piece values. Lets first find the probability
for a Rook to have a safe check for all board places of the King. There are 4 general types
of King places. A King in the corner, a King in the rectangular shape defined by
(b2,c2,...h2,i2) x (b2,b3,...,b7), a King on (b8 or c8 or ....h8 or i8) or (b1 or c1 or ...
h1 or i1) and a King in all other squares. Every square in each category
has the same properties with each other of the category, when we are speaking about calculating the wanted probability, so we
just have to deal for doing our calculations, with any single random square that belongs to
this category and that will represent all others.
A King in the corner. We choose a8 square to represent all others in the category. So the Rook has 8(horizontal) + 6(vertical) safe checks.
So there are 14 safe checks when the King is on a8. So as there are 4 squares in this category we have 4·14 = 56 safe checks
for this category.
A King in the rectangular shape defined by (b2,c2,...h2,i2) x (b2,b3,...,b7).
We choose b2 square to represent all others in the category. So the Rook has 5(vertical) + 7(horizontal) = 12
safe checks when the King is on b2. But there are 8·6 = 48 such squares. So we have a total of 12·48 = 576
safe checks for this category.
A King in (b8 or c8 or ....h8 or i8) or (b1 or c1 or ...
h1 or i1). We choose b8 square to represent all others in the category.
So the Rook has 7(horizontal) + 6(vertical) = 13 safe checks when the King is on b8.
But there are 8·2 = 16 such squares. So we have a total of 16·13 = 208
safe checks for this category.
A King in all other squares.We choose a2 square to represent all others in the category.
So the Rook has 8(horizontal) + 5(vertical) = 13 safe checks when the King is on a2.
But there are 6·2 = 12 such squares. So we have a total of 12·13 = 156
safe checks for this category.
So we have a total of 56 + 576 + 208 + 156 = 996 safe checks for all King positions.
The number of possible arrangements(counting the illegal too) of Rook and King is:
80·79 = 6320 as we have 80 available squares for the King to place and 79 remaining for the Rook, so 80·79 for both.
So we have a probability of having a safe check to the King,
when the King and the Rook are placed randomly on the 10x8 board alone(counting and the illegal positions), equal to:
996/6320 = 0.1576
Applying the same procedure for the Bishop and the Knight we have:
Safe check probability Knight = 440/6320 = 0.0696
Safe check probability Bishop = 266/((80·40+80·39)/2) = 266/3160 = 532/6320 = 0.0842
And as we found before:Safe check probability Rook = 996/6320 = 0.1576
Now we can easily conclude that the safe check probability for any piece that combines the movement
of 2 others, is the sum of the safe check probabilies of the other 2. So we have:
Safe check probability Archbishop = 972/6320 = 0.1538
Safe check probability Queen = 1528/6320 = 0.2418
Safe check probability Chancellor = 1436/6320 = 0.2272
So now when we are going to our DEFINITION-1, we have that the Queen is the most
powerful piece on the board and it is followed by the Chancellor. But to made the values more
readable an understandable we can do the following: We know that a Knight is worth 3 Pawns in Chess.
We find the safe check probability for the Knight at Chess and we find it to be equal with
0.0833. So we compare that number with the safe check probability for the Knight at Gothic Chess.
And we see that we have a decrease of: 100·(0.0833 - 0.0696)/0.0833 = 16.44 %
So now (having in mind that a Knight is worth 3 Pawns in Chess) we can give the value of
3·(1-0.1644) = 2.5 to the Gothic Chess Knight. And since we know that it has a safe check probability
of 0.0696 in Gothic Chess AND also because of our DEFINITION-1 that says that the strength of the pieces has a linear relation
with the safe check probability, we can compare safe check probabilities and conclude the relative strength for each piece relative to the
Knight. And so give to every piece the value for its worth. So we have the table:
| PIECE | VALUE |
|---|
| Pawn | 1.0 |
| Knight | 2.5 |
| Bishop | 3.02 |
| Archbishop | 5.52 |
| Rook | 5.66 |
| Chancellor | 8.16 |
| Queen | 8.68 |
But as we see the Rook is worth more than an Archbishop and also 2 Rooks much more than a Queen.
This for someone who knows Gothic Chess it seems wrong. So it has to be corrected in one way.
Experienced Chess players have modified the standard piece values derived with this method, in order to correct
things that this method underestimates. So they reduce the power of the Rook to be one Pawn less than
the Bishop + Knight. Also they increase the value of Queen by 9/8 to have her be a Pawn up
the sum of Bishop + Rook. Altough there should be many opinions, of what the adjustments must be,
to make it more accurate i will give 3 tables:
Ed Trice's Adjustments:
Rook = 94.5% of the value of the Chess Rook set at 5.00.
Queen = (9/8)·Queen
Archbishop = (9/8)·Archbishop
Chancellor = (9/8)·Chancellor
We have:
| PIECE | VALUE |
|---|
| Pawn | 1.0 |
| Knight | 2.5 |
| Bishop | 3.02 |
| Rook | 4.73 |
| Archbishop | 6.22 |
| Chancellor | 8.13 |
| Queen | 8.73 |
Simplified due to his following words: "In general, you would not want to trade the Archbishop
and the Knight for a Queen, so for this reason, we make one other small adjustment,
then round the numbers off to something reasonable for tournament use" to :
| PIECE | VALUE |
|---|
| Pawn | 1.0 |
| Knight | 2.5 |
| Bishop | 3.0 |
| Rook | 4.75 |
| Archbishop | 6.50 |
| Chancellor | 8.25 |
| Queen | 8.75 |
My adjustments:
Archbishop is worth 1 Pawn more from Bishop + Knight in most cases.
Queen is slighly worse(4%) than Archbishop and Knight in most cases.
Rook is worth around 0.8 Pawns less than the calculated value.
Chancellor is around 12-13% better than the calculated value.
So we have:
| PIECE | VALUE |
|---|
| Pawn | 1.0 |
| Knight | 2.5 |
| Bishop | 3.02 |
| Rook | 4.86 |
| Archbishop | 6.52 |
| Chancellor | 8.28 |
| Queen | 8.66 |
Now lets define another way of measuring procedure that would give us
an approximation of the pieces strength. This has been created as far as i know, by Reinhard Scharnagl and more details
are available clicking here:

DEFINITION-2: The strength of any piece
has a linear relation with the sum of: the
number of all affected squares which could be covered at
all by the chess piece on an empty board, calculated for every possible square it can go(this concerns all ever possible targets of defense or attack) and
the number of all affected directions into which a chess piece can radiate its effect on an empty
board, calculated for every possible square it can go (this concerns the maximum number of figures targetable at the same time), divided by the number of all possible squares the piece can legally go on an empty board.
Let's examine for example the Rook, with this DEFINITION-2.
Then the number of all affected directions into which a chess piece can radiate its effect on an empty
board, calculated for every possible square it can go
(this concerns the maximum number of figures targetable at the same time),
can be easily calculated in the following way:
We take 3 general categories that contain the whole board: The corners,
the rectangular shape defined by (b2,c2,...,h2,i2) x (b2,b3,...,b6,b7) and the other board.
And we examine each case:
The corners. In each square the Rook affects 2 directions. We have 4 corners. So the sum of the affected directions(with the additional aforementioned things
we don't have to refer each time) by the Rook = 2·4 = 8.
The rectangular shape defined by (b2,c2,...,h2,i2) x (b2,b3,...,b6,b7).
In each square it affects 4 directions. We have 48 squares in this case so the sum of the affected directions by the Rook = 4·48 = 192.
The other board. In each square it affects 3 directions. Sum of the affected directions by the Rook = 3·(2·6+2·8) = 84.
So we have a total of 8+192+84 = 284 affected DIRECTIONS by the Rook(with the additional aforementioned things).
To find the number of all affected SQUARES which could be covered at
all by the chess piece on an empty board, calculated for every possible square it can go(this concerns
all ever possible targets of defense or attack) we should do a similar procedure, but this time we don't
have to divide the board into cases as a Rook on each square affects the same number of
squares. And the number is 16.
So we have a total of 16·80 = 1280 affected SQUARES by the Rook(with the additional aforementioned things).
So we have a sum of these 2 numbers equal with 284 + 1280 = 1564.
And now we can
find the number of the DEFINITION-2, that expresses the strength of the pieces in a linear way compared to the other piece's same calculated number.
It is 1564/80 = 19.55 since 80 are the legel squares a Rook can go on an empty board.
And thus we
conclude that the number that characterizes the Rook's strength is 19.55!
Doing a similar procedure for all the other pieces we take the following table:
| PIECE | VALUE |
|---|
| Pawn | 3.60 |
| Knight | 11.00 |
| Bishop | 12.95 |
| Rook | 19.55 |
| Archbishop | 23.95 |
| Chancellor | 30.55 |
| Queen | 32.50 |
| ***King | 13.40 |
So now and since the relation according to DEFINITION-2 between the strength of the pieces is linear, we can simply divide all values by 3.60 to have the Pawn value as our main value of 1.
| PIECE | VALUE |
|---|
| Pawn | 1.0 |
| Knight | 3.05 |
| Bishop | 3.60 |
| Rook | 5.43 |
| Archbishop | 6.65 |
| Chancellor | 8.48 |
| Queen | 9.03 |
| ***King | 3.72 |
And now we make an overview table:
| PIECE | Ed Trice-VALUES | R.Scharnagl-VALUES |
My-VALUES |
|---|
| Pawn | 1.0 | 1.0 | 1.0 |
| Knight | 2.5 | 3.05 | 2.5 |
| Bishop | 3.02 | 3.60 | 3.02 |
| Rook | 4.73 | 5.43 | 4.86 |
| Archbishop | 6.22 | 6.65 | 6.52 |
| Chancellor | 8.13 | 8.48 | 8.28 |
| Queen | 8.73 | 9.03 | 8.66 |
| ***King | ??? | 3.72 | 3.55 (An estimation...) |
We can give endless definitions for measuring procedures that would give us
an approximation of the pieces strength. for example instead of only calculate the
number of safe checks for every piece that can give in an emtpy board with the King, we
can also calculate the number of safe threats a piece can give to all the others when it is on an empty
board with it and
make comparison tables again. Or to combine with the Definition-2 and have other values as well.
It's really a matter of choice what procedure-definition we would choose and one way to see
what is the best choice, is to create Gothic Chess programs that will have the values of each
method and play against each other and see the results............
And of course we have to understand that all these methods for knowing the piece values are
pointless tries, for playing a better game, to cover our incapability of understanding
the game perfectly. Since we can't find the best play, we define such ways of undestanding the game
by patterning as many things possible and give them meanings we can understand....This
works when playing against creatures of similar approach(humans and human's creations=computers),
but it is very inefficient against the best play which is unknown until today.......
Gothic Chess official site:












